Consider the formula of the derivative of the scalar function U in the direction of λ

The second factors are the projections of a single vector directed by the beam λ.

We take the vector, the projections of which on the coordinate axes will be the values \u200b\u200bof private derivatives in the selected T. p (x, y, z).

This vector is called the gradient of the function U (x, y, z) and designate graduili

Definition. The gradient of the function U (x, y, z) is called the vector, the projections of which are values \u200b\u200bof private derivatives of this function, i.e.

The derivative of the function in this direction is equal to the scalar product of the gradient of the function per unit vector of this direction.

Revealing the scalar product, we get

,

where φ is the angle between the vector gradu. and beam λ.

Reaches the greatest value

So, there is the highest value of the derivative in this TR, and the Grad U direction coincides with the direction of the beam leaving from the trial, along which the function changes faster.

We establish the relationship between the direction of the gradient of the function and the surfaces of the scalar field.

Theorem. The gradient of the function u (x, y, z) at each point coincides with the normal to the surface of the scalar field level passing through this point.

Evidence. Choose an arbitrary T. P 0 (X 0, Y 0, Z 0).

Surface equation

level passing through

t. Will u (x, y, z) \u003d,

u 0 \u003d U (x 0, y 0, z 0)

The equation is normal to this surface in T., there will be

From here it follows that the guide vector of normal, having projections is a gradient of the function U (x, y, z) in t. P 0, bt.d.

Thus, the gradient at each point is perpendicular to the tangent plane to the surface of the level passing through this point, i.e. His projection on this plane is zero.

Hence: The derivative in any direction taking into account the surface of the level passing through this point is zero.

The main properties of the gradient function:

2) Grad. where with - Const.

4) Grad.

All properties are proved using the definition of a gradient function.

Example. In t. M (1, 1, 1) find the direction of the greatest change in the scalar field and the magnitude of this change.

Concept derivative in direction It is considered for the functions of two and three variables. To understand the meaning of the derivative in the direction, you need to compare derivatives by definition

Hence,

Now we can find a derivative in the direction of this function according to its formula:

And now - homework. It is given a function of not three, but only two variables, but a few otherwise the guide vector is specified. So you have to repeat again vector algebra .

Example 2.Find a derivative function at point M.0 (1; 2) in the direction of the vector where M.1 - point with coordinates (3; 0).

The vector defining the direction of the derivative may be given in this form, as in the following example - as decomposition by orthop coordinate axes But this is a good familiar theme from the very beginning of the vector algebra.

Example 3.Find a derivative function At point M.0 (1; 1; 1) In the direction of the vector.

Decision. Find the guide cosines vector

Find private derivatives at the point M.0 :

Therefore, we can find a derivative in the direction of this function according to its formula:

.

Gradient Function

Gradient function multiple variables at point M.0 characterizes the direction of maximum growth of this function at the point M.0 and the magnitude of this maximum growth.

How to find a gradient?

Need to determine vector, projections of which on the axis of coordinates are values private derivatives ,, this feature at the appropriate point:

.

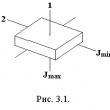
That is, it should be presentation of vector over orthop coordinate axes In which the private derivative is multiplied by each ORT.

1 0 The gradient is directed along the normal to the level surface (or to the level line, if the Flat field).

2 0 The gradient is aimed at an increase in the field function.

3 0 The gradient module is equal to the highest derivative in the direction of the field:

These properties give the invariant characteristics of the gradient. They suggest that the GraduTu vector indicates the direction and magnitude of the greatest changes in the scalar field at this point.

Note 2.1.If the function u (x, y) is a function of two variables, then vector

lies in the Oxy Plane.

Let U \u003d U (X, Y, Z) and V \u003d V (X, Y, Z) differically differentiated at the point m 0 (x, y, z) of the function. Then the following equalities take place:

a) grad () \u003d; b) Grad (UV) \u003d Vgradu + Ugradv;

c) GRAD (U V) \u003d Gradu Gradv; d) g) grad \u003d, v;

e) gradu (\u003d gradu, where, u \u003d u () has a derivative of software.

Example 2.1.The function u \u003d x 2 + y 2 + z 2 is given. Determine the gradient of the function at the point M (-2; 3; 4).

Decision. According to formula (2.2) we have

The surfaces of the level of this scalar field are the family of spheres x 2 + y 2 + z 2, the vector gradu \u003d (- 4; 6; 8) is a normal vector of planes.

Example 2.2.Find the gradient of the scalar field U \u003d x-2y + 3z.

Decision. According to formula (2.2) we have

The surfaces of the level of this scalar field are planes

x-2y + 3z \u003d C; Vector gradu \u003d (1; -2; 3) There is a normal vector of the planes of this family.

Example 2.3. Find the largest steepness of the surface of the surface u \u003d x y at the point M (2; 2; 4).

Decision. We have:

Example 2.4. Find a single vector of normal to the surface of the level of the scalar field U \u003d x 2 + Y 2 + Z 2.

Decision.Surface levels of this scalar field-sphere x 2 + y 2 + Z 2 \u003d C (C\u003e 0).

The gradient is directed by normal to the surface of the level, so

Determines the vector of normal to the surface of the level at the point M (x, y, z). For single normal vector we obtain expression

Example 2.5. Find the gradient of the field u \u003d, where and permanent vectors, R -Dius vector point.

Decision. Let be

Then:. According to the rule of differentiation of the determinant get

Hence,

Example 2.6.Find a distance gradient, where p (x, y, z) is the studied point of the field, P 0 (x 0, y 0, z 0) is some fixed point.

Decision. We have - single direction vector.

Example 2.7. Find the angle between the gradients of the functions at the point M 0 (1,1).

Decision. We find the gradients of these functions at the point M 0 (1,1), we have

; The angle between Gradu and Gradv at the point M 0 is determined from equality

Hence \u003d 0.

Example 2.8. Find a derivative direction, radius - vector is equal

Decision.We find the gradient of this function:

Substituting (2.5) in (2.4), we get

Example 2.9. Find at point M 0 (1; 1; 1) The direction of the greatest change in the scalar field U \u003d XY + YZ + XZ and the magnitude of this largest change at this point.

Decision. The direction of the greatest changes in the field is indicated by the GRAD U (M) vector. Find it:

And, it means. This vector defines the direction of the greatest increase in this field at the point M 0 (1; 1; 1). The magnitude of the greatest change in the field at this point is

Example 3.1.Find vector line vector fields where -tranny vector.

Decision.We have so that

Multiply the numerator and denominator of the first fraction on x, the second one, the third-on Z and lay down. Using the proportion property, we get

Hence xdx + ydy + zdz \u003d 0, which means

x 2 + Y 2 + Z 2 \u003d A 1, A 1 -CONST\u003e 0. Multiplying now the numerator and denominator of the first fraction (3.3) on C 1, the second one - with 2, the third one with 3 and folding the metering, we get

Where from 1 DX + C 2 DY + C 3 DZ \u003d 0

And, therefore, with 1 x + C 2 y + C 3 z \u003d a 2. A 2 -CONST.

The desired equations of vector lines

These equations show that vector lines are obtained as a result of the intersection of areas with a common center at the beginning of the coordinates, with planes perpendicular to the vector. From here it follows that the vector lines are circles, the centers of which are located on a straight line passing through the origin in the direction of the vector p. The planes of the circles are perpendicular to the specified direct.

Example 3.2.Find a vector line of fields passing through the point (1.0.0).

Decision. Differential equations vector lines

From here we have. Solving the first equation. Or if you enter the parameter T, then in this case the equation takes the form or DZ \u003d BDT, from where Z \u003d BT + C 2.

Lecture 15. "Differentiation of the function of several variables"

Gradient function of two variables and derivative in the direction.

Definition. Gradient function

called vector

.

As can be seen from the determination of the function gradient, the components of the gradient vector are private derivatives.

Example. Calculate the function gradient

at point A (2,3).

Decision. Calculate private derivatives.

In general, the quality gradient is:

=

We substitute the coordinates of the point A (2,3) in the expressions of private derivatives

The gradient of the function at a point A (2,3) has the form:

Similarly, it is possible to determine the concept of the gradient of the functions of the three variables:

Definition. The gradient of the function from three variables

called vector

Otherwise, this vector can be recorded as follows:

Definition derivative in direction.

Let the function of two variables be specified

and arbitrary vector

Consider the increment of this function taken along this vector

Those. Vector collinear in relation to the vector. The length of the increment of argument

The derivative in some direction is called the limit of the relation of the increment of the function along this direction for the length of the argument increment, when the length of the argument is striving for 0.

Formula for calculating the derivative in the direction.

Based on the definition of the gradient, the derivative function in the direction can be calculated as follows.

some vector. Vector with the same direction but single Call lengths

The coordinates of this vector are calculated as follows:

From the determination of the derivative direction, the derivative in the direction can be calculated according to the following formula:

The right side of this formula is a scalar product of two vectors.

Therefore, the derivative in the direction can be represented as the following formula:

From this formula, there are several important properties of the gradient vector.

The first property of the gradient follows from the obvious fact that the scalar product of two vectors takes the greatest value when the vector coincide in the direction. The second property follows from the fact that the scalar product of perpendicular vectors is zero. In addition, from the first property follows the geometric meaning of the gradient - the gradient is a vector, along the direction that the derivative of the highest direction. Since the derivative in the direction determines the tangent of the tilt angle to the surface of the function, the gradient is directed along the greatest tilt tongue.

Example 2. For function (from Example 1)

Calculate the derivative in the direction

at point A (2,3).

Decision. To calculate the derivative in the direction, it is necessary to calculate the gradient vector at the specified point and the unit direction vector (i.e. normalize vector).

The gradient vector was calculated in Example 1:

Calculate a single direction vector:

Calculate the derivative in the direction:

# 2. Maximum and minimum functions of several variables.

Definition. Function

Has a maximum at the point (i.e., and), if

Definition. Quite similarly say that the function

Has a minimum at the point (i.e., and), if

for all points, close enough to the point and different from it.

The maximum and minimum of the functions are called the extremums of the function, i.e. it is said that the function has an extremum at a given point if this function has a maximum or at least at the point.

For example, a function

It has an obvious minimum z \u003d -1 at x \u003d 1 and y \u003d 2.

It has a maximum at the point at x \u003d 0 and y \u003d 0.

Theorem. (The necessary conditions of extremum).

If the function reaches an extremum at, then each particular derivative of the first order from Z or turns into zero at these values \u200b\u200bof the arguments, or does not exist.

Comment. This theorem is not sufficient to study the question of the extreme values \u200b\u200bof the function. Examples of functions can be given that at some points have zero private derivatives, but does not have an extremum in these point.

Example. Functions that have zero private derivatives, but does not have an extremum.

Indeed:

Sufficient extremum conditions.

Theorem. Let in some region containing a point, the function has continuous private derivatives to third order inclusive; Let, in addition, the point is a critical function of the function, i.e.

Then when

Example 3.2. Explore the maximum and minimum function

We will find critical points, i.e. The points in which the first private derivatives are equal to zero or do not exist.

First, calculate the private derivatives themselves.

Equate private derivatives zero and solve the following system of linear equations

We multiply the second equation for 2 and fold with the first. It turns out the equation only from Y.

We find and substitute in the first equation

Transform

Consequently, the point () is critical.

We calculate the second derivatives of the second order and substitute the coordinates of the critical point in them.

In our case, it is not necessary to substitute the values \u200b\u200bof critical points, since the second derivatives are numbers.

As a result, we have:

Consequently, the critical point found is an extremum point. Moreover, since

then this point is minimum.

Brief theory

The gradient is called the vector, the direction of which indicates the direction of the maximum rapid increase in the function f (x). Finding this vector value is associated with the definition of private derived functions. The derivative in the direction is a scalar value and shows the rate of change of function when driving along the direction specified by some vector.

An example of solving the problem

The task

Danies feature, point and vector. To find:

The solution of the problem

Finding a gradient function

1) We will find the gradient of the function at the point:

The desired gradient:

Finding a derivative in the direction of the vector

2) Find a derivative in the direction of the vector:

where-China, formed by vector and axis

The desired derivative at the point:

The price strongly affects the urgency of the solution (from day to several hours). Online assistance on the exam / standings is carried out by appointment.

The application can be left directly in the chat, having previously throwing the condition of tasks and informing the decision you need. Answer time - a few minutes.